What's the secret to solving inequalities in algebra?

Are you looking forward to driving a car?

The law states that you need to be at least 16 years old to drive a car.

The law doesn't say you have to be exactly 16.

Photo by S. Widua on Unsplash

In math, an exact amount comes from an equation — but if the amount has a range of possible values, this is called an inequality.

There are inequalities everywhere in the real world. Mathematics is just a way to model and describe the real world.

Can you write this inequality mathematically?

We can say that, x = the age at which you can drive a car.

Then x is greater (>) or equal (=) to 16: x ≥ 16

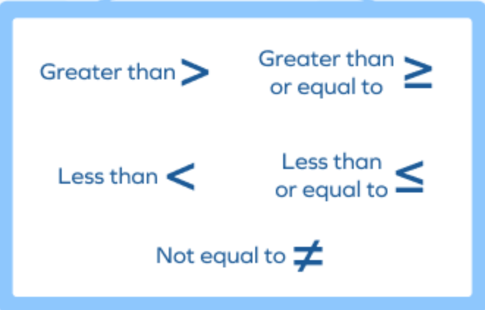
What is an inequality?

An inequality is a comparison between two values, numbers, or expressions that aren't equal (but possibly equal).

There are 5 inequality symbols to remember and use:

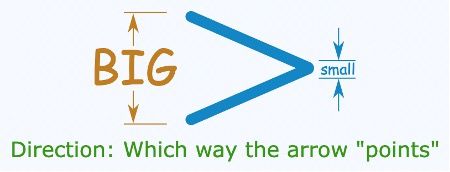
How do we solve algebraic inequalities?

We solve inequalities in the exact same way as equations — but equations have one answer, and an inequality has a range of solutions.

When calculating the values of the inequality, we have to pay attention to the direction of the inequality.

Let's look at the rules to solve algebraic inequalities using addition, subtraction, multiplication, and division.

Let's start with algebraic inequalities using addition.

x + 9 is greater than or equal to 6.

x + 9 ≥ 6

If we're solving for x by itself, we want to get rid of that 9 next to it, so we subtract 9 from both sides.

x + 9 – 9 ≥ 6 – 9

This gives us our answer.

x is greater than or equal to negative 3.

x ≥ -3

Here's an example:

x minus 4 is less than 8.

x – 4 < 8

First, we're going to add 4 to both sides.

x – 4 + 4 < 8 + 4

Then we simplify.

x < 12

It’s as simple as that!

There's a special rule for multiplication and division.

When you multiply or divide by a negative number, you have to flip your sign in the opposite direction.

If you are multiplying or dividing by a positive number, don’t worry about this step.

Let’s look at an example:

Negative 5x is greater than 15.

-5x > 15

To get x by itself, we need to divide both sides by negative 5.

Remember, since we are dividing by -5, we have to flip our inequality sign!

So x is less than negative 3.

x < -3 

x over 7 is less than or equal to 4x.

x/7 ≤ 4x

For this inequality, we need to multiply both sides by 7.

When solving inequalities with multiplication and division, do we flip our sign?

No, we don’t have to since we're multiplying by a positive number.

We’ll multiply both sides by 7, and then we get x, which is less than or equal to 28.

7(x/7) ≤ (7) 4x

x ≤ 28

Photo by Tim Mossholder on Unsplash

Solving inequalities: question #1

The number X could be 8 or any number greater than 8.

How could we write this mathematically?

Select one of the following answers:

A: x ≥ 8 B: x = 8 C: x < 8 D: x ≤ 8

The correct answer is: A

Why? Inequality sign ≥ indicating greater or equal to

Solving inequalities: question #2

Raymond had 5 candy bars (x). Emily ate some of his candy bars. How many candy bars does Raymond have now?

How could write this mathematically?

Select one of the following answers:

A: x ≥ 5 B: x = 5 C: x < 5 D: x ≤ 5

The correct answer is: C

Why? Inequality sign < indicating less than

Solving inequalities: question #3

Solve the following inequality for y.

- 5y < 30

Select one of the following answers:

A: y < 6 B: y ≤ 6 C: y ≤ - 6 D: y < - 6

The correct answer is: D

Why? Divide both sides with -5 to get y on its own.

Photo by Fabian Møller on Unsplash

Solving inequalities: question #4

Solve the following inequality for y.

2y + 3 ≥ y – 7

Select one of the following answers:

A: 10 ≤ y B: -10 ≤ y C: y ≤ - 5 D: y < 5

The correct answer is: B

Did you get that last answer?

If not....let's give it a try together

Add 7 to both ends of the equation:

2y + 3 + 7 ≥ y – 7 + 7 to get 2y + 10 ≥ y

Now, subtract 2y from each side:

2y - 2y + 10 ≥ y - 2y to get 10y ≥ -y

Divide by negative 1 and flip the sign to get your final answer:

-10 ≤ x

Let's try an everyday inequality problem:

Peter has $25 to buy nuts and berries.

• Nuts cost $5 per kilogram. Peter buys 3 kilograms of nuts

• Berries cost $7 for a kilogram. How many kilograms does Peter buy?

Which of the following inequalities can be used to find c? Choose your answer:

A. 15 + 7c ≥ 25

B. 15 + 7c ≤ 25

C. 5 + 7c ≥ 25

D. 5 + 7c ≤ 25

You can see that solving inequalities in real life is very useful!

Need more exercises or examples?

Try these links.